
An Arabian Knight
| 1920/08/22 | ||
| Film | 50 min | |
| ACT ADV FAN | USA | |
A young Egyptian goes to the rescue of his employers, a wealthy European family, when they are menaced by a local strongman and his gang. Japanese actor Sessue Hayakawa is cast as an ancient Egyptian donkey boy in An Arabian Knight. The humble Hayakawa rescues high-born Lillian Hall from lascivious pasha Fred Jones. All this brouhaha is actually a dream experienced by Hall. In the tradition of the "flashback" sequences in the like-vintage Cecil B. DeMille productions, Hall is able to apply the events of the dream into her contemporary circumstances.

Archer's Paradox
An archer must, in order to hit his target, not aim directly at it, but slightly to the side.
| S - | Ep - | 1913// |
| High | - min | |
The term archer's paradox refers to the phenomenon of an arrow traveling in the direction it is pointed at at full draw, when it seems that the arrow would need to pass through the starting position it was in before being drawn, where it was pointed to the side of the target. The bending of the arrow (around the bow), when released, is the explanation of the paradox and should not be confused with the paradox itself. Flexing of the arrow when shot from a modern 'center shot' bow is still present and is caused by a variety of factors, mainly the way the string is deflected from the fingers as the arrow is loosed. The term was first used by E.J. Rendtroff in 1913, and as understanding was gained about the arrow flexing out of the way of the bow as it is shot (as first filmed by Clarence Hickman) and then experiencing oscillating back-and-forth bending as it travels toward the target,[4] this dynamic flexing has incorrectly become a common usage of the term, causing misunderstanding by those only familiar with modern target bows, which being 'centre shot' do not actually show any paradoxical behaviour as the arrow is always pointing visually along its line of flight.
Archie (1943)
Time Trouble
| S - | Ep 7 | 1944/03/ |
| Comic | - min | |

Archie Superhero Comics
Archie Superhero Comics
| S - | Ep - | |
| Comic | - min | |

Archie's Weird Mysteries
Archie's Date With Fate
| S 1 | Ep 34 | 2000/02/14 |
| TV Series Episode | - min | |
When a thief steals a time travel device from Dilton's lab, Archie finds himself trapped in a time loop. He relives the same horrible day over and over again, always ending with the same disastrous date with Veronica. Archie must use Dilton's unstable prototype time travel belt to stop the thief responsible for his plight. Will he ever escape his date with fate?

Archie's Weird Mysteries
Alternate Riverdales
| S 1 | Ep 35 | 2000/02/15 |
| TV Series Episode | - min | |
A time traveler from the future, with a vendetta against Archie, changes Riverdale's past to alter Archie's present. Archie soon finds himself traveling through a series of wild alternate Riverdales. In one, big Ethel has a crush on Dilton. In another, Jughead is a vegetarian and even in one where Veronica is the sweet girl-next-door and Betty is the spoiled rich girl. Archie must once again wear the time belt to stop his enemy from making the cruelest change of all in Riverdale's past - preventing Archie's parents from meeting so that Archie will never be born.

Archie's Weird Mysteries
Teen Out Of Time
| S 1 | Ep 36 | 2000/02/16 |
| TV Series Episode | - min | |
Archie's time-traveling enemy once again menaces Riverdale with a monster from prehistoric times while Archie is trapped in Riverdale's past. Using the unreliable time belt, Archie manages to jump backward in time, visiting key points in Riverdale history. Each time Archie uses the time belt, he gets closer and closer to the present - but will he get back in time to save his friends from becoming dinosaur chow?

Archimedes Paradox (aka the Hydrostatic Paradox)
A massive battleship can float in a few litres of water.
| S - | Ep - | |
| High | - min | |
The Archimedes paradox, named after Archimedes of Syracuse, or the hydrostatic paradox, states that an object can float in a quantity of water that has less volume than the object itself, if its average density is less than that of water. A more general formulation of the paradox is that "that any quantity of water, or other fluid, how small whatsoever, may be made to balance and support any quantity, or any weight, how great whatsoever". The implication of this is that a large, massive object can float in a relatively small volume of liquid, provided that it is surrounded by it. One extreme application of the paradox is that a battleship can float in a few buckets of water, provided that the water surrounds the hull completely and that the ship would have floated had it been in open water.

Are You Afraid of the Dark?
The Tale of the Hungry Hounds
| S 1 | Ep 5 | 1992/09/12 |
| TV Series Episode | 30 min | |
Two girls go to an attic where one of them tries on a uniform that belonged to a dead relative where her spirit lives on.

Are You Afraid of the Dark?
The Tale of Locker 22
| S 2 | Ep 3 | 1993/07/03 |
| TV Series Episode | 30 min | |
A young French immigrant having trouble at her new school, uncovers a mystery about a girl, the former occupant of her school locker, who died in 1968.

Are You Afraid of the Dark?
The Tale of the Carved Stone
| S 3 | Ep 7 | 1994/02/26 |
| TV Series Episode | 30 min | |
A new girl in town trying to makes friends buys a magic stone, which causes her to go back in time to the 1890s to meet another lonely child who lived in the house.

Are You Afraid of the Dark?
The Tale of the Long Ago Locket
| S 4 | Ep 2 | 1994/10/08 |
| TV Series Episode | 30 min | |
A boy is transported by to the Revolutionary War, where he must help a solider return to his girlfriend, before she marries another man.

Are You Afraid of the Dark?
The Tale Of A Door Unlocked
| S 5 | Ep 10 | 1996/01/27 |
| TV Series Episode | 22 min NR | |
| DRA FAM FAN | ||
Sardo sells a magic door to a teenage boy where once he opens the door, the future happens in front of him.

Are You Afraid of the Dark?
The Tale of the Time Trap
| S 7 | Ep 8 | 2000/05/07 |
| TV Series Episode | 30 min | |
Jason buys an ancient 'Persian victory box' from Sardo's Magic Shop which houses a genie who grants Jason's every wish - with dire consequences.

Are You Afraid of the Dark?
The Tale of the Night Nurse
| S 7 | Ep 13 | 2000/06/11 |
| TV Series Episode | - min | |
A murdering nurse's ghost begins terrorizing two teen girls when they spend the week at their grandfather's house.

Arena
| Film | - min | |

Arga: La prophetie
Prince Argai
| S 1 | Ep 1 | 2000/09/02 |
| TV Series Episode | 24 min | |
| ADV SF | France | |
New York 2075. The evil Queen Dark rules the world and by casting a spell upon girls she remains alive forever. Prince Argai from 1250 a.d. is furious when he discovers his fiancee Angel has been enchanted; a lightning flash sends him to 2075 where he seeks refuge with private detective Oscar Lightbulb, his assistant Barnaby and their secretary Miss Moon. It is discovered that a book of prophecies exists that details every ingredient they need for the potion to rescue Angel. They copy this book, but it gets stolen by Queen Dark who then knows their every next move. Will they succeed in collecting the ingredients, rescuing Angel and the destruction of Queen Dark?

Ariana's Quest
| Film | - min | |

"Aristotle and the Gun"
| S - | Ep - | 1958/02/ |
| Short Story | - min | |
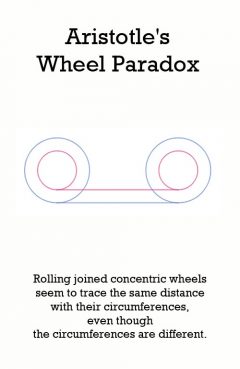
Aristotle's Wheel Paradox
Rolling joined concentric wheels seem to trace the same distance with their circumferences, even though the circumferences are different.
| S - | Ep - | |
| - min | ||
Aristotle's wheel paradox is a paradox from the Greek work Mechanica traditionally attributed to Aristotle. There are two wheels, one within the other, whose rims take the shape of two circles with different diameters. The wheels roll without slipping for a full revolution. The paths traced by the bottoms of the wheels are straight lines, which are apparently the wheels' circumferences. But the two lines have the same length, so the wheels must have the same circumference, contradicting the assumption that they have different sizes: a paradox. The fallacy is the assumption that the smaller wheel indeed traces out its circumference, without ensuring that it, too, rolls without slipping on a fixed surface. In fact, it is impossible for both wheels to perform such motion. Physically, if two joined concentric wheels with different radii were rolled along parallel lines then at least one would slip; if a system of cogs were used to prevent slippage then the wheels would jam. A modern approximation of such an experiment is often performed by car drivers who park too close to a curb. The car's outer tire rolls without slipping on the road surface while the inner hubcap both rolls and slips across the curb; the slipping is evidenced by a screeching noise. Alternatively, the fallacy is the assumption that the smaller wheel is independent of the larger wheel. Imagine a tire as the larger wheel, and imagine the smaller wheel as the interior circumference of the tire and not as the rim. The movement of the inner circle is dependent on the larger circle. Thus its movement from any point to another can be calculated by using an inverse of their ratio.