Release Message:  Hot water can, under certain conditions, freeze faster than cold water, even though it must pass the lower temperature on the way to freezing. Authored by Erasto Mpemba.

Description:  The observation that, in some circumstances, warmer water can freeze faster than colder water. Although there is evidence of the effect, there is disagreement on exactly what the effect is and under what circumstances it occurs. There have been reports of similar phenomena since ancient times, although with insufficient detail for the claims to be replicated. A number of possible explanations for the effect have been proposed. Further investigations will need to decide on a precise definition of "freezing" and control a vast number of starting parameters in order to confirm or explain the effect.

Release Message:  A mouse on the loose in the penthouse makes Peabody (Chris Parnell)'s skin crawl. The inventor of the world's first submarine sets out to find a sea monster.

Description:  Mouse Hunt: Peabody and Sherman have cleaned the penthouse from top to bottom, but a mouse is soon found running about, making Peabody's skin crawl. David Bushnell: Peabody and Sherman join David Bushnell, the inventor of the submarine, on an undersea journey to find a sea monster. Historical Figures: Joseph Lister, Saint Patrick, David Bushnell, George Washington

Release Message:  Anything that can go wrong will go wrong.

Description:  A law of lawlessness: Anything that can go wrong will go wrong.

Release Message:  A solution concept of a non-cooperative game involving two or more players, in which each player is assumed to know the equilibrium strategies of the other players, and no player has anything to gain by changing only their own strategy unilaterally.

Description:  In game theory, the Nash equilibrium is a solution concept of a non-cooperative game involving two or more players, in which each player is assumed to know the equilibrium strategies of the other players, and no player has anything to gain by changing only their own strategy unilaterally. If each player has chosen a strategy and no player can benefit by changing strategies while the other players keep theirs unchanged, then the current set of strategy choices and the corresponding payoffs constitute a Nash equilibrium. Stated simply, Amy and Wili are in Nash equilibrium if Amy is making the best decision she can, taking into account Wili's decision, and Wili is making the best decision he can, taking into account Amy's decision. Likewise, a group of players are in Nash equilibrium if each one is making the best decision that he or she can, taking into account the decisions of the others.

Release Message:  Increased navigational precision may result in increased collision risk.

Description:  In the case of ships and aircraft, the advent of Global Positioning System (GPS) navigation has enabled craft to follow navigational paths with such greater precision (often on the order of plus or minus 2 meters), that, without better distribution of routes, coordination between neighboring craft and collision avoidance procedures, the likelihood of two craft occupying the same space on the shortest distance line between two navigational points has increased.

Release Message:  A wager between two people seems to favor them both.

Description:  The necktie paradox is a puzzle or paradox within the subjectivistic interpretation of probability theory. It is a variation (and historically, the origin) of the two-envelope paradox. Two men are each given a necktie by their respective wives as a Christmas present. Over drinks they start arguing over who has the cheaper necktie. They agree to have a wager over it. They will consult their wives and find out which necktie is more expensive. The terms of the bet are that the man with the more expensive necktie has to give it to the other as the prize. The first man reasons as follows: winning and losing are equally likely. If I lose, then I lose the value of my necktie. But if I win, then I win more than the value of my necktie. Therefore the wager is to my advantage. The second man can consider the wager in exactly the same way; thus, paradoxically, it seems both men have the advantage in the bet. This is obviously not possible. The paradox can be resolved by giving more careful consideration to what is lost in one scenario ("the value of my necktie") and what is won in the other ("more than the value of my necktie"). If we assume for simplicity that the only possible necktie prices are $20 and $30, and that a man has equal chances of having a $20 or $30 necktie, then four outcomes (all equally likely) are possible:

Release Message:  Adding a new state or voting block might increase the number of votes of another.

Description:  Adding a new state or voting block might increase the number of votes of another.

Release Message:  How do you play a game against an omniscient opponent? Authored by William Newcomb.

Description:  Newcomb's Paradox (Newcomb's Problem) A person is playing a game operated by the Predictor, an entity somehow presented as being exceptionally skilled at predicting people's actions. The exact nature of the Predictor varies between retellings of the paradox. Some assume that the character always has a reputation for being completely infallible and incapable of error; others assume that the predictor has a very low error rate. The Predictor can be presented as a psychic, as a superintelligent alien, as a deity, as a brain-scanning computer, etc. However, the original discussion by Nozick says only that the Predictor's predictions are "almost certainly" correct, and also specifies that "what you actually decide to do is not part of the explanation of why he made the prediction he made". With this original version of the problem, some of the discussion below is inapplicable.

Release Message:  If truth does not exist, the statement "truth does not exist" is a truth, thereby proving itself incorrect.

Description:  If truth does not exist, the statement "truth does not exist" is a truth, thereby proving itself incorrect.

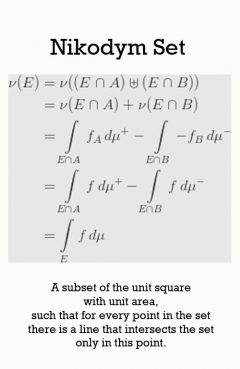
Release Message:  A set contained in and with the same Lebesgue measure as the unit square, yet for every one of its points there is a straight line intersecting the Nikodym set only in that point. Authored by Otto M. Nikodym.

Description:  In mathematics, a Nikodym set is the seemingly paradoxical result of a construction in measure theory. A Nikodym set in the unit square S in the Euclidean plane E2 is a subset N of S such that the area (i.e. two-dimensional Lebesgue measure) of N is 1; for every point x of N, there is a straight line through x that meets N only at x. The existence of such a set as N was first proved in 1927 by the Polish mathematician Otto M. Nikodym. Nikodym sets are closely related to Kakeya sets (also known as Besicovitch sets).

Release Message:  You can have three dice, called A, B, and C, such that A is likely to win in a roll against B, B is likely to win in a roll against C, and C is likely to win in a roll against A.

Description:  A set of dice is nontransitive if it contains three dice, A, B, and C, with the property that A rolls higher than B more than half the time, and B rolls higher than C more than half the time, but it's not true that A rolls higher than C more than half the time. In other words, a set of dice is nontransitive if its "rolls a higher number than more than half the time" relation is not transitive.

Release Message:  If an event exists that would cause a paradox or any "change" to the past whatsoever, then the probability of that event is zero.

Description:  The Novikov self-consistency principle, also known as the Novikov self-consistency conjecture, is a principle developed by Russian physicist Igor Dmitriyevich Novikov in the mid-1980s. Novikov intended it to solve the problem of paradoxes in time travel, which is theoretically permitted in certain solutions of general relativity that contain what are known as closed timelike curves. The principle asserts that if an event exists that would cause a paradox or any "change" to the past whatsoever, then the probability of that event is zero. It would thus be impossible to create time paradoxes.

Release Message:  The outcome of an event or experiment is influenced by the presence of the observer. Authored by William Labov.

Description:  The outcome of an event or experiment is influenced by the presence of the observer.

Release Message:  Why is the night sky black if there is an infinity of stars? Authored by Heinrich Wilhelm Olbers.

Description:  The paradox is that a static, infinitely old universe with an infinite number of stars distributed in an infinitely large space would be bright rather than dark. In astrophysics and physical cosmology, Olbers' paradox, named after the German astronomer Heinrich Wilhelm Olbers (1758-1840) and also called the "dark night sky paradox", is the argument that the darkness of the night sky conflicts with the assumption of an infinite and eternal static universe. The darkness of the night sky is one of the pieces of evidence for a non-static universe such as the Big Bang model. If the universe is static and populated by an infinite number of stars, any sight line from Earth must end at the (very bright) surface of a star, so the night sky should be completely bright. This contradicts the observed darkness of the night. Edward Robert Harrison's Darkness at Night: A Riddle of the Universe (1987) gives an account of the dark night sky paradox, seen as a problem in the history of science. According to Harrison, the first to conceive of anything like the paradox was Thomas Digges, who was also the first to expound the Copernican system in English and also postulated an infinite universe with infinitely many stars.[2] Kepler also posed the problem in 1610, and the paradox took its mature form in the 18th century work of Halley and Cheseaux.[3] The paradox is commonly attributed to the German amateur astronomer Heinrich Wilhelm Olbers, who described it in 1823, but Harrison shows convincingly that Olbers was far from the first to pose the problem, nor was his thinking about it particularly valuable. Harrison argues that the first to set out a satisfactory resolution of the paradox was Lord Kelvin, in a little known 1901 paper,[4] and that Edgar Allan Poe's essay Eureka (1848) curiously anticipated some qualitative aspects of Kelvin's argument

Release Message:  Can an omnipotent being create a rock too heavy for itself to lift? Authored by AverroÔs and St. Thomas Aquinas.

Description:  Can an omnipotent being create a rock too heavy for itself to lift?

Release Message:  It is opposite day today.

Description:  Opposite Day is an unofficial holiday, commonly observed whenever it is declared, where every action is modified so that meaning is negated. Once Opposite Day is declared, statements mean the opposite of what they usually mean. Usually, a person would say, "After this phrase is over, it will be officially opposite day," and then Opposite Day will be officially started. Opposite Day can also be declared retroactively to indicate that what was just asserted had the opposite meaning of what was originally intended (For example when Kipley[when?] was told she was awesome by her awesome friend on opposite day, she did not realize that it was opposite day and was delighted, only to check her calendar seconds later to find out that it was in fact opposite day, which then lead her to go into a deep depression for the next week, illustrating Kipley's moody and depressing nature) (similar to the practice of crossed fingers to automatically nullify promises). Play has been compared to a children's "philosophy course",[1] and the game has been used as an educational aid and suggested as preparation for "standardized testing".[citation needed]

Release Message:  Alpha ignored the man approaching him and treated him as a stranger. The man was his father. Did Alpha ignore his own father and treat him as a stranger?

Description:  Alpha ignored the man approaching him and treated him as a stranger. The man was his father. Did Alpha ignore his own father and treat him as a stranger?

Release Message:  Rigid-body dynamics with contact and friction is inconsistent.

Description:  Painleve Paradox The Painlev e paradox is a well-known example by Paul Painlev in rigid-body dynamics that showed that rigid-body dynamics with both contact friction and Coulomb friction is inconsistent. This is due to a number of discontinuities in the behavior of rigid bodies and the discontinuities inherent in the Coulomb friction law, especially when dealing with large coefficients of friction.[1] There exist, however, simple examples which prove that the Painlev paradoxes can appear even for small, realistic friction. Modeling rigid bodies and friction greatly simplifies such applications as animation, robotics and bio-mechanics, it is only an approximation to a full elastic model requiring complex systems of partial differential equations. Rigid body assumption also allows one to clarify many features that would otherwise remain hidden: Painlev paradoxes are one of them. Moreover the rigid body models can be reliably and efficiently simulated, avoiding stiff problems and issues related to the estimation of compliant contact/impact models, which is often quite a delicate matter. The paradox was mathematically resolved in the 1990s by David E. Stewart....

Release Message:  A gun that has characteristics of both (smoothbore) shotguns and rifles.

Description:  A gun that has characteristics of both (smoothbore) shotguns and rifles.

Release Message:  At the beginning of the 21st century, the US is as powerful as no nation before it, yet as dependent on the global community as never before.

Description:  At the beginning of the 21st century, the US is as powerful as no nation before it, yet as dependent on the global community as never before.