Nikodym Set
Release Date: //1927
Country of Release:
Length:
MPAA:
Medium: Paradox
Genre:
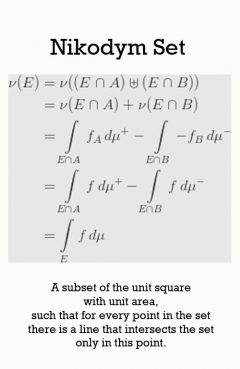
Release Message: A set contained in and with the same Lebesgue measure as the unit square, yet for every one of its points there is a straight line intersecting the Nikodym set only in that point. Authored by Otto M. Nikodym.
Description: In mathematics, a Nikodym set is the seemingly paradoxical result of a construction in measure theory. A Nikodym set in the unit square S in the Euclidean plane E2 is a subset N of S such that the area (i.e. two-dimensional Lebesgue measure) of N is 1; for every point x of N, there is a straight line through x that meets N only at x. The existence of such a set as N was first proved in 1927 by the Polish mathematician Otto M. Nikodym. Nikodym sets are closely related to Kakeya sets (also known as Besicovitch sets).