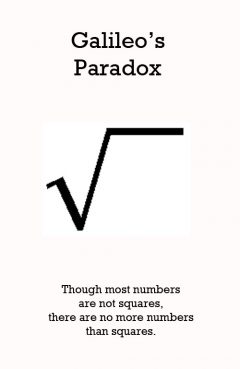
Release Message:  Though most numbers are not squares, there are no more numbers than squares. Authored by Galileo Galilei.

Description:  Galileo's paradox is a demonstration of one of the surprising properties of infinite sets. In his final scientific work, Two New Sciences, Galileo Galilei made apparently contradictory statements about the positive integers. First, some numbers are squares, while others are not; therefore, all the numbers, including both squares and non-squares, must be more numerous than just the squares. And yet, for every square there is exactly one positive number that is its square root, and for every number there is exactly one square; hence, there cannot be more of one than of the other. This is an early use, though not the first, of the idea of one-to-one correspondence in the context of infinite sets. Galileo concluded that the ideas of less, equal, and greater apply to finite sets, but not to infinite sets. In the nineteenth century, using the same methods, Cantor showed that this restriction is not necessary. It is possible to define comparisons amongst infinite sets in a meaningful way (by which definition the two sets he considers, integers and squares, have "the same size"), and that by this definition some infinite sets are strictly larger than others. It is nonetheless remarkable the extent by which Galileo anticipated later work on infinite numbers. He showed that the number of points in a line segment is the same as the number in a larger line segment, but of course he didn't discover Cantor's proof that this is greater than the number of integers. Galileo had more urgent matters in mind. He was addressing the contradictions in Zeno's paradoxes in order to open the way for his mathematical theory of motion.[1] (Naive set theory)